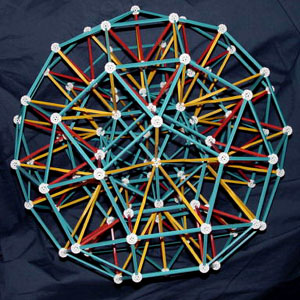
Even with two views, this model is much easier to fathom if you see it in person. Unfortunately, it requires more Zometool pieces than a sane person is likely to have (180 blue, 60 yellow, 60 red, 92 vertices). The outer blue solid is the rhombicosidodecahedron. Inside it are a blue dodecahedron and a blue icosahedron. You can imagine collapsing the rhombicosidodecahedron onto either of the inner solids. For instance, you can slide each of the twenty triangles along the yellow edges down to the twenty triangular faces of the icosahedron: the model contains twenty blue and yellow triangular prisms corresponding to this motion, as well as twelve blue and yellow pentagonal pyramids which show how each pentagon in the rhombicosidodecahedron collapses to a vertex of the icosahedron. The red edges give a similar collapse to the dodecahedron, with a corresponding twelve pentagonal prisms and twenty triangular pyramids.

If you prefer, you may instead focus on the thirty squares in the rhombicosidodecahedron. There are two ways in which to simultaneously collapse all of these squares into line segments, and each is manifested in a family of triangular prisms in the model. Following the red and blue triangular prisms gives the edges of the dodecahedron, while following the yellow and blue triangular prisms gives the edges of the icosahedron.

Note that the icosahedron and dodecahedron are in the standard dual-polyhedron configuration, although the icosahedron is a little smaller than the one you get by connecting the centers of the faces of the dodecahedron.