
These are also pictures of models from the origami display that I installed in the Amherst College Mathematics and Computer Science Department in 1995. However, these models were removed a couple of years after the original installation and before I took any photographs.



While origami is beautiful and versatile art form, it does have its shortcomings as a means to represent polyhedral forms. For example, if you compare the hanging models from the principal exhibit with the polyhedra they represent, you see that the origami models that contain pentagons and hexagons either use support struts to divide these polygons into triangles or are unstable enough to warp easily.
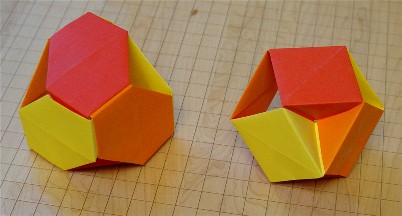
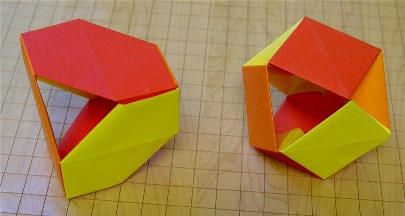
However, there are some cases in which the origami modules themselves cast light on polyhedral relationships. If you try to make a tetrahedron out of Tomoko Fuse's Plain Open Frame Unit (the unit used for six of the nine hanging solids), the normally angled modules lie flat and you get the model on the left of the photographs below, which is a cube with three corners removed. This coincidence reflects the intimate connections between the tetrahedron, octahedron, and cube described here and here. A simple variation in creases gives the model on the right, which (with the eight triangular sides filled in) is a cuboctahedron.