Soap Film Geometry
Minimal Surfaces and Isoperimetric Problems in Mathematics
Summary: If you dip a bent circle of wire into soapy water and pull
it out, there will be a soap film stretching across it. Physically,
this film seeks to have the smallest possible area as a way of
minimizing a certain type of energy. The mathematical study of surfaces
that have minimum area has developed into a rich theory with many
topics of current research. This page is intended to be readable by
nonmathematicians.
 In 1829, the Belgian professor of Physics and Anatomy
Joseph Plateau permanently damaged his eyes in an optical experiment
that involved staring at the sun for twenty-five seconds. At the same
time, he became interested in the physical and geometrical properties
of soap film surfaces, which are elastic in the sense that they have
the smallest possible area. Despite being completely blind by 1843,
Plateau continued his research with help from his family and fellow
scientists, and published many papers on the subject. Meanwhile, a
mathematical theory of surfaces that minimize their area had begun over
the course of the previous century, when the mathematicians Bernoulli,
Euler, and Lagrange developed the "Calculus of Variations." A
mathematical interest renewed by Plateau's experiments led to the
formulation of the "Plateau Problems." For example, we may pose the
following question: "Start with a circle of wire that has been twisted,
bent, and stretched into some new shape. If we dip it into soapy water
and pull it out again, what is the shape of the soap film that
results?" Physically, surface tension makes the resulting soap film
minimize its area while still spanning the wire frame. The analogous
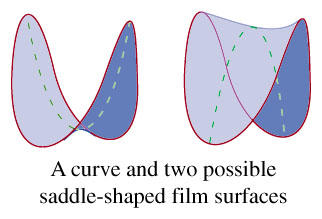
mathematical Plateau Problem is as follows: "You are given a bent
circular curve in three dimensional space, like the wire figure. There
are many different possible two-dimensional surfaces touching the
entire given curve, like attached sheets of plastic wrap. Your task is
to find the one that has the smallest total area." In the 1930s, Jesse
Douglas and Tibor Radó finally showed mathematically that no
matter what shape the curve has, there is always a least-area stretched
disk spanning the curve. Further research, including work in the field
of Geometric Measure Theory, showed that there is always a least area
surface spanning the curve. Today research on various geometrical
aspects of such surfaces continues across the country, often with the
help of experimental equipment consisting of wire and soap water. For
instance, in 1976 Jean Taylor proved that soap film surfaces can only
intersect in two ways: three surfaces can intersect along a curve,
meeting at equal angles of 120 degrees, or four surfaces can intersect
at a point, meeting at about 109 degrees. This property was originally
conjectured by Plateau himself.
In 1829, the Belgian professor of Physics and Anatomy
Joseph Plateau permanently damaged his eyes in an optical experiment
that involved staring at the sun for twenty-five seconds. At the same
time, he became interested in the physical and geometrical properties
of soap film surfaces, which are elastic in the sense that they have
the smallest possible area. Despite being completely blind by 1843,
Plateau continued his research with help from his family and fellow
scientists, and published many papers on the subject. Meanwhile, a
mathematical theory of surfaces that minimize their area had begun over
the course of the previous century, when the mathematicians Bernoulli,
Euler, and Lagrange developed the "Calculus of Variations." A
mathematical interest renewed by Plateau's experiments led to the
formulation of the "Plateau Problems." For example, we may pose the
following question: "Start with a circle of wire that has been twisted,
bent, and stretched into some new shape. If we dip it into soapy water
and pull it out again, what is the shape of the soap film that
results?" Physically, surface tension makes the resulting soap film
minimize its area while still spanning the wire frame. The analogous
mathematical Plateau Problem is as follows: "You are given a bent
circular curve in three dimensional space, like the wire figure. There
are many different possible two-dimensional surfaces touching the
entire given curve, like attached sheets of plastic wrap. Your task is
to find the one that has the smallest total area." In the 1930s, Jesse
Douglas and Tibor Radó finally showed mathematically that no
matter what shape the curve has, there is always a least-area stretched
disk spanning the curve. Further research, including work in the field
of Geometric Measure Theory, showed that there is always a least area
surface spanning the curve. Today research on various geometrical
aspects of such surfaces continues across the country, often with the
help of experimental equipment consisting of wire and soap water. For
instance, in 1976 Jean Taylor proved that soap film surfaces can only
intersect in two ways: three surfaces can intersect along a curve,
meeting at equal angles of 120 degrees, or four surfaces can intersect
at a point, meeting at about 109 degrees. This property was originally
conjectured by Plateau himself. 
Before we can attempt to
solve the Plateau Problem
mathematically, we need precise definitions of concepts such as curve,
surface, and area. The best definitions here would require a fair
amount of calculus, and would lead us to some very interesting
mathematical theories, including Einstein's relativity. Instead, let us
consider some examples. Lines, circles, and the path traveled by a
housefly are examples of curves. A circle is called a "closed curve"
because a little person walking along it would return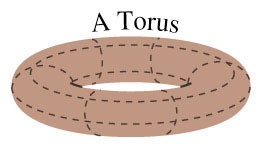 to the place she
started. An important tool of calculus allows us to define a unique
line that is tangent to a curve at any point. For example, a horizontal
line is tangent to the top of a circle. Two-dimensional planes and
spheres are examples of surfaces, as is a horse's saddle and a torus,
which you can think of as the outer surface of a donut. The
sphere and torus are both
examples of "closed surfaces," since they don't have boundary curves.
At a point of a surface, there are lots of different lines that are
tangent to the surface, but there can only be one tangent plane. For
instance, a horizontal plane is tangent to the north pole of a sphere.
to the place she
started. An important tool of calculus allows us to define a unique
line that is tangent to a curve at any point. For example, a horizontal
line is tangent to the top of a circle. Two-dimensional planes and
spheres are examples of surfaces, as is a horse's saddle and a torus,
which you can think of as the outer surface of a donut. The
sphere and torus are both
examples of "closed surfaces," since they don't have boundary curves.
At a point of a surface, there are lots of different lines that are
tangent to the surface, but there can only be one tangent plane. For
instance, a horizontal plane is tangent to the north pole of a sphere. 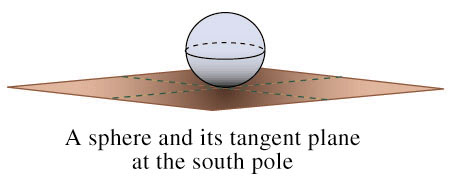
We may formulate a one-dimensional
version of the Plateau problem as follows: "Given two points in space,
find the curve connecting them which has the least distance. The
solution, of course, is always a straight line segment." In attacking
the two-dimensional problem, our intuition is that an area-minimizing
surface should be as "flat" as possible, with no unnecessary hills or
valleys. For instance, if we dip a perfectly circular wire into soapy
water, the resulting soap film should be a flat disc spanning the
circle. However, if our wire boundary is bent, then we expect that the
surface with least area that spans it will have to bend some. The
mathematical concept of curvature is a way to describe the
bending of a surface.
First, we can define this
property for a curve by considering what happens to a tiny ant that
walks along the curve with constant speed one centimeter per second.
Its velocity, which tells us how fast it's going and in what direction,
always points along the line tangent to the curve. We can imagine this
as an arrow pointing out of the ant's head. The acceleration of the ant
tells us how fast its velocity arrow is changing and in what direction.
This arrow points in the direction the curve is bending, and is
perpendicular to the tangent line. We call the ant's acceleration the
"curvature vector" of the curve. For example, a line has zero curvature
since its tangent line never changes, while a circle has a curvature of
constant length pointing toward its center. To ex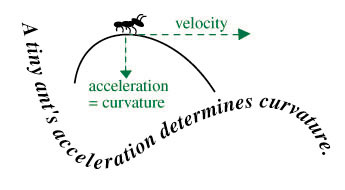 amine the
geometry of
a surface, we again place an ant and watch it move at constant
speed. But this time it can go in many
different directions. So, there are many different kinds of curvature
for surfaces. The most important one in the theory of soap films is the
mean curvature, which is the average of the biggest (most
positive) and smallest (most negative) curvatures of curves on the
surface. Let us consider the example of a sphere, with the convention
that the "up" direction from the surface points toward the center (we
may think of an ant inside a round balloon). Then a point on the sphere
will have a positive number for its mean curvature, because the surface
curves upward in every direction. On the other hand, a saddle-shaped
surface might have zero mean curvature where the rider sits, because in
the minimal direction it curves downward (where the rider's legs might
go), while in the maximal direction it curves upward (along the horse's
spine). What do you think we can say about the mean curvature on a
torus? An important mathematical theorem is this one: "If a
two-dimensional surface spanning a boundary curve minimizes area
compared to others spanning the same boundary, then this surface has
zero mean curvature at every point." You can imagine for instance that
if the sur
amine the
geometry of
a surface, we again place an ant and watch it move at constant
speed. But this time it can go in many
different directions. So, there are many different kinds of curvature
for surfaces. The most important one in the theory of soap films is the
mean curvature, which is the average of the biggest (most
positive) and smallest (most negative) curvatures of curves on the
surface. Let us consider the example of a sphere, with the convention
that the "up" direction from the surface points toward the center (we
may think of an ant inside a round balloon). Then a point on the sphere
will have a positive number for its mean curvature, because the surface
curves upward in every direction. On the other hand, a saddle-shaped
surface might have zero mean curvature where the rider sits, because in
the minimal direction it curves downward (where the rider's legs might
go), while in the maximal direction it curves upward (along the horse's
spine). What do you think we can say about the mean curvature on a
torus? An important mathematical theorem is this one: "If a
two-dimensional surface spanning a boundary curve minimizes area
compared to others spanning the same boundary, then this surface has
zero mean curvature at every point." You can imagine for instance that
if the sur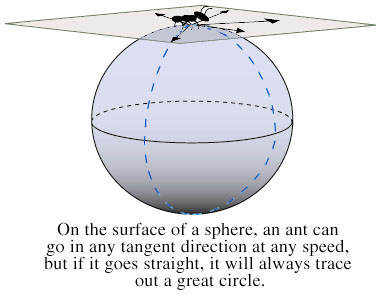 face had a mountain (with negative
mean curvature), then a
surface with the mountain cut off would have less area. The precise
mathematical proof can be found in the references below. Notice that
the implication here does not mean that every surface with zero mean
curvature minimizes area. However, mathematicians call any surface with
zero mean curvature a minimal surface.
face had a mountain (with negative
mean curvature), then a
surface with the mountain cut off would have less area. The precise
mathematical proof can be found in the references below. Notice that
the implication here does not mean that every surface with zero mean
curvature minimizes area. However, mathematicians call any surface with
zero mean curvature a minimal surface.
There are many interesting and
still unsolved problems in the theory of minimal surfaces. For example,
one can find a curve in space which is the boundary curve of several
different minimal surfaces. It is unknown whether there is a closed
curve in space bounding an infinite number of minimal surfaces. A large
amount of current research concerns the idea of minimal surfaces in
higher dimensions. I have become interested in general geometric
properties of higher dimensional minimal surfaces, such as how smooth
or pointy they are.
A slightly different unsolved
problem involves soap bubbles rather than films. It is known that a
spherical soap bubble is a surface that minimizes its area, subject to
the restriction that it encloses a fixed volume. This mathematical fact
was conjectured in ancient Greece. Now consider the problem of two
bubbles. What is the best surface to enclose and sep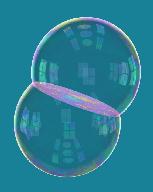 arate two different
volumes? By experimenting with bubbles, we can find a nice surface that
should work, but how can we prove that it is the best? This question
remained open until just recently, and it spurred much interesting
research in an area known as Geometric Measure Theory. In 1996, a pair
of mathematicians solved the case in which the two
bubbles have equal volume, using a computer. In 1993, a group of
undergraduate researchers succeeded in one lower dimension. That is,
they showed that the standard double circle in the plane separating two
regions of given area uniquely minimizes its perimeter. The
three-dimensional problem was solved by Hutchings, Morgan, Ritore and
Ros in 2000. The analogous problem for the triple bubble is wide open,
and the two-dimensional version was the subject of a very recent PhD thesis.
arate two different
volumes? By experimenting with bubbles, we can find a nice surface that
should work, but how can we prove that it is the best? This question
remained open until just recently, and it spurred much interesting
research in an area known as Geometric Measure Theory. In 1996, a pair
of mathematicians solved the case in which the two
bubbles have equal volume, using a computer. In 1993, a group of
undergraduate researchers succeeded in one lower dimension. That is,
they showed that the standard double circle in the plane separating two
regions of given area uniquely minimizes its perimeter. The
three-dimensional problem was solved by Hutchings, Morgan, Ritore and
Ros in 2000. The analogous problem for the triple bubble is wide open,
and the two-dimensional version was the subject of a very recent PhD thesis.
Here is a practical version of the
soap bubble problem that often appears
in calculus classes: "A soup company wants to package its soup
efficiently
using cylindrical cans. The cans must be made to contain 16 cubic
inches
of volume. The top and bottom discs of the can cost fifteen cents per
square inch to make, while the side costs twelve cents per square inch.
What is the cheapest cylindrical can?"
References:
Colding, T. and Minicozzi, W, Minimal Surfaces.
Courant Institute Lecture Notes 4, 1999.
Foisy, Alfaro, Brock, Hodges, and Zimba, "The
Standard Soap Bubble in R2 Uniquely Minimizes Perimeter", Pacific
Journal of Mathematics 159 (1993) p.47-59.
''Proof of the
Double Bubble Conjecture'', by Michael Hutchings, Frank Morgan,
Manuel Ritoré and Antonio Ros
Isenberg, Cyril, The science of soap films and soap
bubbles. Tieto Ltd., Clevedon, 1978.
Morgan, Frank, Riemannian Geometry: a Beginner's
Guide. Second edition. A K Peters, Wellesley, 1998.
Morgan, Frank, "Minimal Surfaces, Crystals,
Shortest Networks, and Undergraduate Research", Mathematical
Intelligencer 14 (1992) no. 3 p.37-44.
Morgan, Frank, "Mathematicians, Including
Undergraduates, Look at Soap Bubbles", American Mathematical Monthly
101 (1994) no. 4 p.343-351.
(The problem of infinitely many minimal surfaces was addressed by a
high school student in a letter to the author.)
Morgan, Frank, Geometric measure theory. A
beginner's guide. Third edition. Academic Press, Inc., San Diego,
CA, 2000.
Taylor, Jean E., "The structure of singularities in
soap-bubble-like and
soap-film-like minimal surfaces". Annals of Mathematics (2) 103 (1976),
no. 3, 489--539.
Internet Links:
A Bubble Recipe:
2/3 cup liquid dishwashing detergent. Add enough water
to make one gallon. For tougher, longer-lasting bubbles, add 1
tablespoon glycerine available in any drugstore.) Note: Bubble solution
improves with age. For best results, allow the mixture to sit in an
open container for at least one day before use.
Copyright: Alex Meadows, 2006
 In 1829, the Belgian professor of Physics and Anatomy
Joseph Plateau permanently damaged his eyes in an optical experiment
that involved staring at the sun for twenty-five seconds. At the same
time, he became interested in the physical and geometrical properties
of soap film surfaces, which are elastic in the sense that they have
the smallest possible area. Despite being completely blind by 1843,
Plateau continued his research with help from his family and fellow
scientists, and published many papers on the subject. Meanwhile, a
mathematical theory of surfaces that minimize their area had begun over
the course of the previous century, when the mathematicians Bernoulli,
Euler, and Lagrange developed the "Calculus of Variations." A
mathematical interest renewed by Plateau's experiments led to the
formulation of the "Plateau Problems." For example, we may pose the
following question: "Start with a circle of wire that has been twisted,
bent, and stretched into some new shape. If we dip it into soapy water
and pull it out again, what is the shape of the soap film that
results?" Physically, surface tension makes the resulting soap film
minimize its area while still spanning the wire frame. The analogous
mathematical Plateau Problem is as follows: "You are given a bent
circular curve in three dimensional space, like the wire figure. There
are many different possible two-dimensional surfaces touching the
entire given curve, like attached sheets of plastic wrap. Your task is
to find the one that has the smallest total area." In the 1930s, Jesse
Douglas and Tibor Radó finally showed mathematically that no
matter what shape the curve has, there is always a least-area stretched
disk spanning the curve. Further research, including work in the field
of Geometric Measure Theory, showed that there is always a least area
surface spanning the curve. Today research on various geometrical
aspects of such surfaces continues across the country, often with the
help of experimental equipment consisting of wire and soap water. For
instance, in 1976 Jean Taylor proved that soap film surfaces can only
intersect in two ways: three surfaces can intersect along a curve,
meeting at equal angles of 120 degrees, or four surfaces can intersect
at a point, meeting at about 109 degrees. This property was originally
conjectured by Plateau himself.
In 1829, the Belgian professor of Physics and Anatomy
Joseph Plateau permanently damaged his eyes in an optical experiment
that involved staring at the sun for twenty-five seconds. At the same
time, he became interested in the physical and geometrical properties
of soap film surfaces, which are elastic in the sense that they have
the smallest possible area. Despite being completely blind by 1843,
Plateau continued his research with help from his family and fellow
scientists, and published many papers on the subject. Meanwhile, a
mathematical theory of surfaces that minimize their area had begun over
the course of the previous century, when the mathematicians Bernoulli,
Euler, and Lagrange developed the "Calculus of Variations." A
mathematical interest renewed by Plateau's experiments led to the
formulation of the "Plateau Problems." For example, we may pose the
following question: "Start with a circle of wire that has been twisted,
bent, and stretched into some new shape. If we dip it into soapy water
and pull it out again, what is the shape of the soap film that
results?" Physically, surface tension makes the resulting soap film
minimize its area while still spanning the wire frame. The analogous
mathematical Plateau Problem is as follows: "You are given a bent
circular curve in three dimensional space, like the wire figure. There
are many different possible two-dimensional surfaces touching the
entire given curve, like attached sheets of plastic wrap. Your task is
to find the one that has the smallest total area." In the 1930s, Jesse
Douglas and Tibor Radó finally showed mathematically that no
matter what shape the curve has, there is always a least-area stretched
disk spanning the curve. Further research, including work in the field
of Geometric Measure Theory, showed that there is always a least area
surface spanning the curve. Today research on various geometrical
aspects of such surfaces continues across the country, often with the
help of experimental equipment consisting of wire and soap water. For
instance, in 1976 Jean Taylor proved that soap film surfaces can only
intersect in two ways: three surfaces can intersect along a curve,
meeting at equal angles of 120 degrees, or four surfaces can intersect
at a point, meeting at about 109 degrees. This property was originally
conjectured by Plateau himself. 
 to the place she
started. An important tool of calculus allows us to define a unique
line that is tangent to a curve at any point. For example, a horizontal
line is tangent to the top of a circle. Two-dimensional planes and
spheres are examples of surfaces, as is a horse's saddle and a torus,
which you can think of as the outer surface of a donut. The
sphere and torus are both
examples of "closed surfaces," since they don't have boundary curves.
At a point of a surface, there are lots of different lines that are
tangent to the surface, but there can only be one tangent plane. For
instance, a horizontal plane is tangent to the north pole of a sphere.
to the place she
started. An important tool of calculus allows us to define a unique
line that is tangent to a curve at any point. For example, a horizontal
line is tangent to the top of a circle. Two-dimensional planes and
spheres are examples of surfaces, as is a horse's saddle and a torus,
which you can think of as the outer surface of a donut. The
sphere and torus are both
examples of "closed surfaces," since they don't have boundary curves.
At a point of a surface, there are lots of different lines that are
tangent to the surface, but there can only be one tangent plane. For
instance, a horizontal plane is tangent to the north pole of a sphere. 
 amine the
geometry of
a surface, we again place an ant and watch it move at constant
speed. But this time it can go in many
different directions. So, there are many different kinds of curvature
for surfaces. The most important one in the theory of soap films is the
mean curvature, which is the average of the biggest (most
positive) and smallest (most negative) curvatures of curves on the
surface. Let us consider the example of a sphere, with the convention
that the "up" direction from the surface points toward the center (we
may think of an ant inside a round balloon). Then a point on the sphere
will have a positive number for its mean curvature, because the surface
curves upward in every direction. On the other hand, a saddle-shaped
surface might have zero mean curvature where the rider sits, because in
the minimal direction it curves downward (where the rider's legs might
go), while in the maximal direction it curves upward (along the horse's
spine). What do you think we can say about the mean curvature on a
torus? An important mathematical theorem is this one: "If a
two-dimensional surface spanning a boundary curve minimizes area
compared to others spanning the same boundary, then this surface has
zero mean curvature at every point." You can imagine for instance that
if the sur
amine the
geometry of
a surface, we again place an ant and watch it move at constant
speed. But this time it can go in many
different directions. So, there are many different kinds of curvature
for surfaces. The most important one in the theory of soap films is the
mean curvature, which is the average of the biggest (most
positive) and smallest (most negative) curvatures of curves on the
surface. Let us consider the example of a sphere, with the convention
that the "up" direction from the surface points toward the center (we
may think of an ant inside a round balloon). Then a point on the sphere
will have a positive number for its mean curvature, because the surface
curves upward in every direction. On the other hand, a saddle-shaped
surface might have zero mean curvature where the rider sits, because in
the minimal direction it curves downward (where the rider's legs might
go), while in the maximal direction it curves upward (along the horse's
spine). What do you think we can say about the mean curvature on a
torus? An important mathematical theorem is this one: "If a
two-dimensional surface spanning a boundary curve minimizes area
compared to others spanning the same boundary, then this surface has
zero mean curvature at every point." You can imagine for instance that
if the sur face had a mountain (with negative
mean curvature), then a
surface with the mountain cut off would have less area. The precise
mathematical proof can be found in the references below. Notice that
the implication here does not mean that every surface with zero mean
curvature minimizes area. However, mathematicians call any surface with
zero mean curvature a minimal surface.
face had a mountain (with negative
mean curvature), then a
surface with the mountain cut off would have less area. The precise
mathematical proof can be found in the references below. Notice that
the implication here does not mean that every surface with zero mean
curvature minimizes area. However, mathematicians call any surface with
zero mean curvature a minimal surface.  arate two different
volumes? By experimenting with bubbles, we can find a nice surface that
should work, but how can we prove that it is the best? This question
remained open until just recently, and it spurred much interesting
research in an area known as Geometric Measure Theory. In 1996, a pair
of mathematicians solved the case in which the two
bubbles have equal volume, using a computer. In 1993, a group of
undergraduate researchers succeeded in one lower dimension. That is,
they showed that the standard double circle in the plane separating two
regions of given area uniquely minimizes its perimeter. The
three-dimensional problem was solved by Hutchings, Morgan, Ritore and
Ros in 2000. The analogous problem for the triple bubble is wide open,
and the two-dimensional version was the subject of a very recent PhD thesis.
arate two different
volumes? By experimenting with bubbles, we can find a nice surface that
should work, but how can we prove that it is the best? This question
remained open until just recently, and it spurred much interesting
research in an area known as Geometric Measure Theory. In 1996, a pair
of mathematicians solved the case in which the two
bubbles have equal volume, using a computer. In 1993, a group of
undergraduate researchers succeeded in one lower dimension. That is,
they showed that the standard double circle in the plane separating two
regions of given area uniquely minimizes its perimeter. The
three-dimensional problem was solved by Hutchings, Morgan, Ritore and
Ros in 2000. The analogous problem for the triple bubble is wide open,
and the two-dimensional version was the subject of a very recent PhD thesis.